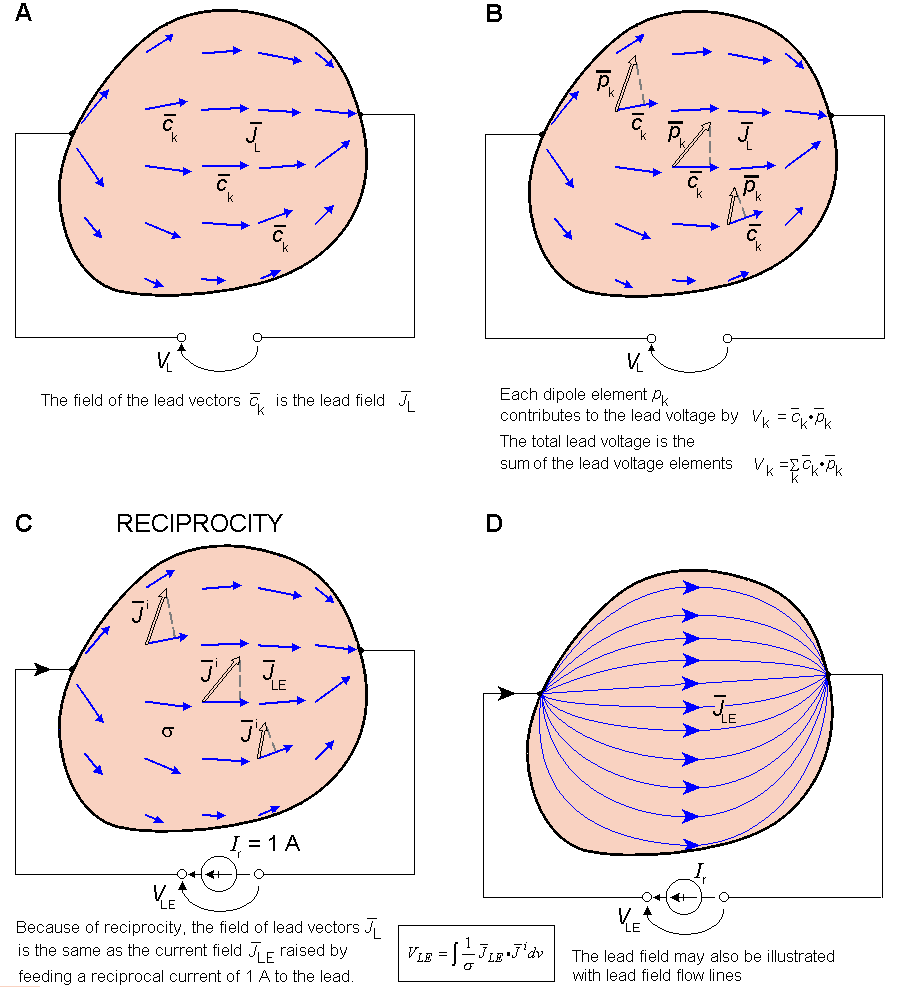
Fig. 11.19. The definition of the lead field and different ways to illustrate it.
(A) When defining the lead field, we assume a fixed electrode pair constituting a lead, and we observe the behavior of the lead vector as a function of the location k of the dipole source within the volume conductor. This field of lead vectors is the lead field _L.
(B) When we know the lead vector at each location k, we obtain the contribution of each dipole element _k to the lead voltage: V_k = _k · _k . Due to superposition, the total lead voltage V_L is the sum of the lead voltage elements.
(C) Based on the reciprocity theorem, the lead field _LE is the same as the electric current field if a (reciprocal) current I _r of 1 A is introduced to the lead. The lead voltage due to a volume source of distribution ⁱ is obtained through integrating the dot product of the lead field current density and the source density throughout the volume source.
(D) The lead field may also be illustrated with the lead field current flow lines.